Blog

What is Welfare Economics?

Welfare economics is the study of how the allocation of resources and goods affects social welfare. This relates directly to the study of economic efficiency and income distribution, as well as how these two factors affect the overall well-being of people in the economy.

Welfare economics is normative in nature. By evaluating the social efficiency of a particular policy, normative economics or welfare economics prescribes a policy option that is economically as well as socially desirable. There is a possibility of welfare losses which is undesirable and desirable welfare gains due to allocation of resources or policy changes.

This welfare loss or gain is the outcome of interdependence among various economic agents of an economy. For example, a change in the price of one factor can easily change the price of other factors. This interrelationship among various parts of the economy means that certain particular change in one part of the economy affects the resource allocation in all other parts of it.

Therefore, when one’s welfare increases, it may decrease the welfare of others which leads to economic inefficiency in any change in the allocation of resources or goods. In this context, Italian economist Vilfredo Pareto (1848-1923) laid down three marginal conditions so that economic efficiency can be achieved.

According to Pareto for the attainment of economic efficiency the marginal conditions to be satisfied in an economy are:

1. Efficiency in the allocation of distribution of goods (Consumption efficiency).
   
2. Efficiency in allocation of resources (Product efficiency).
   
3. Efficiency in the allocation of product mix (Simultaneous efficiency of production and consumption).

Pigovian Welfare Economics

According to Pigou, ‘The Economics of Welfare’ is very pragmatic. Welfare depends upon one’s state of mind regarding the satisfaction or utilities received from his consumption. Individuals’ welfare is determined on the basis of the extent to which an individual’s wants are satisfied.

He limits his explanation only to that part of social welfare that can be brought directly or indirectly into a relationship with the measuring rod of money. This is called economic welfare, i.e., welfare or satisfaction derived by an individual from the use of exchangeable goods and services.

It means that he talks only about economic welfare. And so he says economic welfare is a part of total or general welfare and is a function of goods and their relative prices. Social welfare is calculated simply by summing up all individuals welfare.

But he failed to take into account other forms of welfare, which depends on many other elements like the working condition, security, housing status and human relationship.

Welfare Conditions

Pigou adopts dual criteria for judging the changes happening in social welfare. They are called dual conditions for maximum social welfare. They are as follows:

Maximization of National Income

Maximization of national income: According to Pigou, if national income increases without a corresponding change in the supply of factors, then there will be an increase in social welfare.

Transfer of National Income from the Rich to the Poor

Transfer of National Income from the Rich to the Poor: Welfare is maximum when the share of the poor increases without affecting adversely the total income or the given national income.

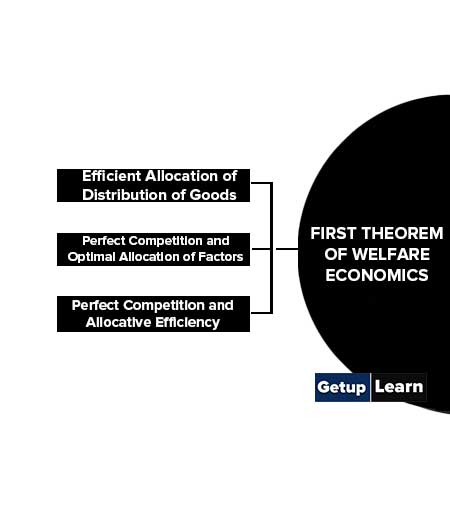
First Theorem of Welfare Economics

We know that Pareto efficiency has three marginal conditions:

1. Efficient Allocation of Distribution of Goods
2. Perfect Competition and Optimal Allocation of Factors between Firms
3. Perfect Competition and Allocative Efficiency

Efficient Allocation of Distribution of Goods

With regard to the distribution of goods among the consumers, one important marginal condition of Pareto efficiency is the marginal rate of substitution (MRS) which is equal for all the consumers. To prove it, let us assume an economy under the following conditions:

• Two consumers A and B along with their budget constraint/limit are always trying to maximize their level of satisfaction from the consumption of goods X and Y.
  
  
• There exists perfect competition so that the price of goods X and Y are given and the same for both consumers A and B.

Perfect Competition and Optimal Allocation of Factors between Firms

The marginal condition for Pareto optimality relates to the optimal allocation of factors in the production of various goods. To achieve this condition, the marginal rate of technical substitution (MRTS) of factors must be equal among the firms.

Perfect Competition and Allocative Efficiency (Optimum Direction of Production)

This condition requires the equality between MRT (Marginal Rate of Product Transformation) in production and MRS in consumption for every pair of commodities and for individuals. In the case of a very simple economy composed of only two commodities X and Y and two individuals A and B, Pareto optimality requires that:

MRTxy = MRSxyA = MRSxyB

Again, this condition can be ensured under perfect competition. We know a firm under perfect competition in equilibrium point produces output to that extent where its marginal cost is equal to the price of the commodity.

Thus, for firms that are in perfect competition, MCX=PX and MCY=PY where MCX and MCY marginal costs of production of commodities X and Y respectively and PX and PY are prices of commodities X and Y. Therefore, in perfect competition, the equilibrium will set at such quantities as:

MCx/ MCy = Px/ Py

Second Theorem of Welfare Economics

The second welfare theorem tells us that when indifference curves are convex to their origin, every efficient allocation at any point on the contract curve represents competitive equilibrium depending on the initial allocation of an endowment.

At competitive equilibrium, a suitable redistribution of endowments can ensure social welfare in an economy.

The case where the second welfare theorem does not hold

In the case of the non-convexity of preferences, the second welfare theorem does not hold. For example, out of two individuals, individual A has non-convex preferences. Then point like E can never be competitive market equilibrium.

E represents Pareto efficiency because here MRSxy A=MRSxy B on indifference curve Ia of individual A and IB of individual B. But at point E there is no set of prices at which both A and B will simultaneously want to consume. Suppose if we assumed that price set as a slope of the common tangent at E.Then individual B would prefer combinations of two goods X and Y at point E where his highest possible indifference is tangent to the budget line. A, on the other hand, due to non-convex preferences choose bundle T on higher indifference curve IA1 instead of bundle E on indifference curve IA.